I’m going to say it: problem-solving in math can be difficult.
Consider walking into your classroom an hour from now and launching into a problem-solving lesson for your elementary kiddos. What is your knee-jerk reaction? What kinds of emotions are stirred up at the thought? I’m willing to bet that you aren’t exactly grinning from ear to ear or jumping for joy.
Problem-solving in math is often a topic that we educators tend to tiptoe around until absolutely necessary; after all, the topic doesn’t exactly evoke positive feelings. Problem-solving is, frankly, hard to teach, hard to learn, and is very often misunderstood. In addition, the subject is riddled with unwarranted and erroneous beliefs about the nature, role, and process of problem-solving due in large part to prior math anxiety and feelings of inadequacy. Taken altogether, the nature of these factors reveals the pièce de résistance of the problem-solving struggle: flawed methodology.
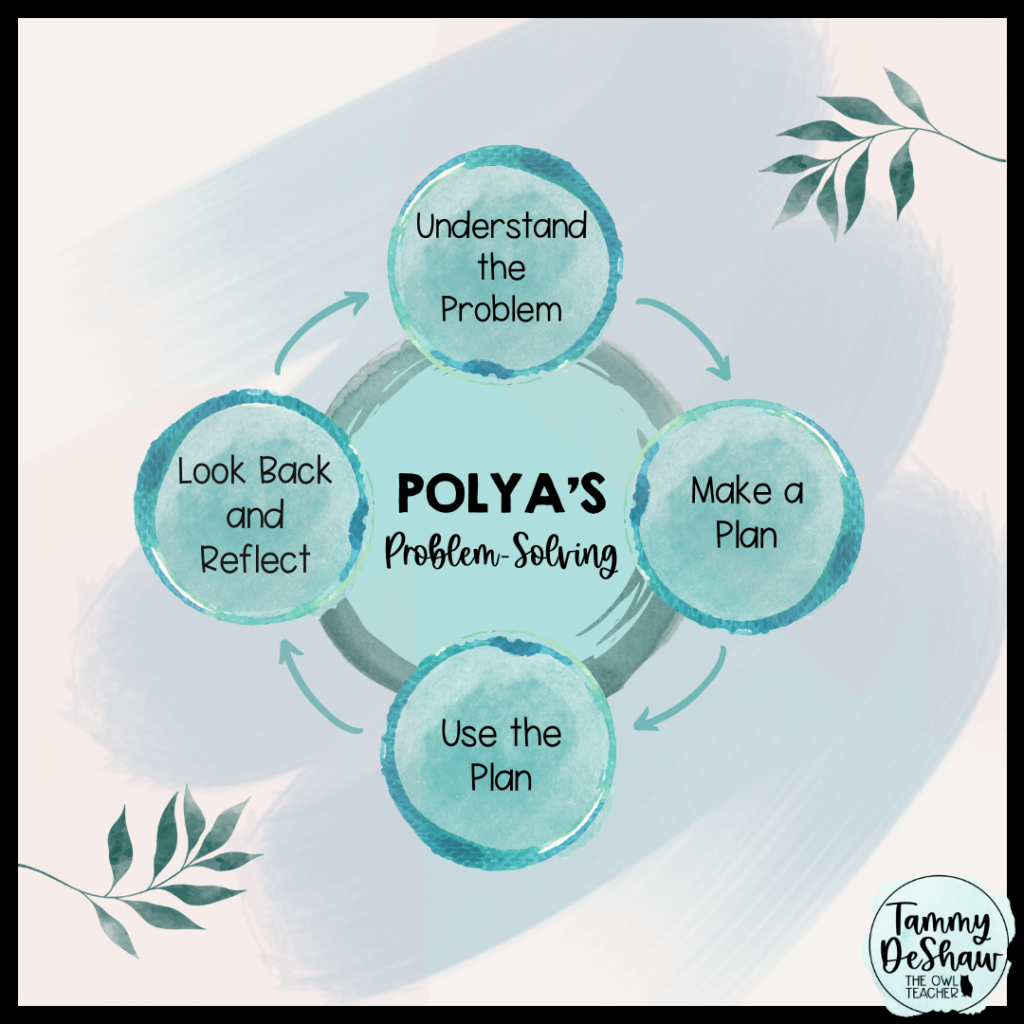
Traditionally, most schools have taught problem-solving according to the recommended strategies from dusty old textbooks. For decades, we’ve been led to believe that the subject is best taught with Polya’s four-step method (who doesn’t love a good four-step model foldable?), then effectively taught a vast array of strategies: working backward, making a table, logical reasoning, and so on. To our students, we provide a view that word problems are nothing more than cleverly disguised computational exercises that can be easily solved using “keywords” or by simply identifying the operation at work. Is that ringing any bells?
Great–now put those bells away. It’s time to rehash those old ways of thinking.
The WRONG Way to Teach Problem-Solving
Teaching Problem-Solving as a Separate Topic
While we do see word problems slapped on at the bottom of worksheets or at the end of pages of a lesson, it seems more common to find an entire page dedicated to a specific strategy at the tail end of a chapter (though these pages are often separate and can be difficult to reconcile with the overarching lesson). Some classrooms have a dedicated day of the week for problem-solving or have even created a separate unit entirely for the subject. Oh, and who among us isn’t familiar with the popular “problem of the day” activities?
Using Word Problems as a Trojan Horse
We all know the story of the Trojan horse used to win the city of Troy. Whether intentional or not, many teachers tend to teach problem-solving through word problems, like a modern-day spin on the hollow horse of Greece. By hiding problem-solving in these word problem strategies, it begins to seem like teaching problem-solving can only be done through word problems!
Every time I see a textbook or worksheet related to the subject, the first thing I notice are the word problems offered at the bottom to help children problem-solve. I know that some sheets bring diversity in the form of sudoku puzzles, logic puzzles, and other math puzzles to help build critical thinking, but it is not quite the same. Further, these puzzles are often not provided with any meaningful context, and can even be more confusing if describing places, things, people, or anything unfamiliar.
Teaching Heuristics and Strategies via Prescribed Drill and Practice
Wait, Tammy… Are you throwing shade on the Polya model?!
Well… Yes and no. Problem-solving heuristics such as Polya’s “read, plan, solve, check” model are still ideal concepts for broaching the subject. The various strategies (trial and error, finding patterns, etc.) can be beneficial, too! By no means am I telling you to forget everything you learned in college–after all, I’m an elementary teacher, not a tenured professor. However, these concepts are not the end-all-be-all of problem-solving. In fact, I would warn fellow educators against relying solely on these ideals, especially when used in conjunction with any drill-and-practice methods.
When we provide children with a systematic approach to problem-solving, even indirectly, it does little to improve their ability to solve math problems in general. Students will generally memorize the strategy for solving a problem, leading to a shallow and thoughtless understanding of how the problem and solution interact. Children are more hung up on which strategy to use as well as when and how to use the strategy that they lose focus on the math part. In other words, there is no real learning–only surface-level memorization of whichever strategies will help them pass the test.
The RIGHT Way to Teach Problem-Solving
The world is new to our little ones. This wide-eyed curiosity makes students natural problem solvers! When we allow children to explore things on their own and are encouraged with an inquiry-based approach, we are influencing brain development and improving their performance.
The benefits of student-led exploration are part of what makes concepts like STEM invaluable to children and educators alike and are a huge reason why I push scaffolding over rescuing as often as I do. When students are given the tools to explore and inquire, their natural tendency is to satisfy their curiosity–they want to learn! If we rescue students or provide them with answers immediately, their development is stifled. On that note, let’s dive into ways to nurture students’ growth rather than impede it!
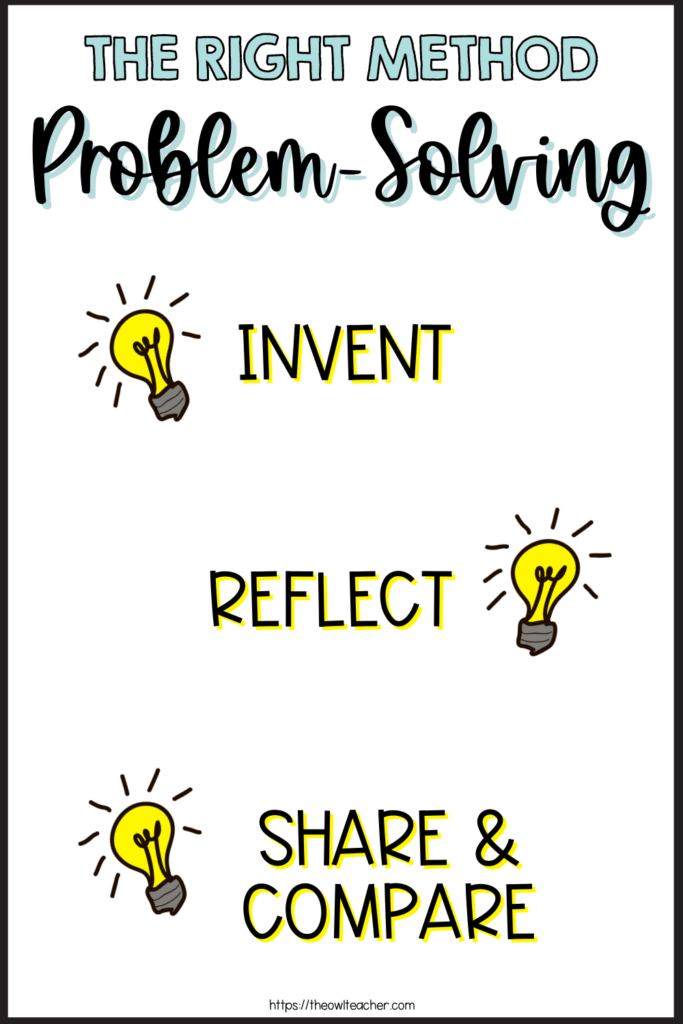
Allow Children to Invent their Own Strategies
Let students be free to explore, investigate, struggle, and learn from one another. Encourage children to solve problems in ways that make sense to them. I know that may mean watching them create methods that are unusual, creative, or confusing; however, you are also giving children the opportunity to take ownership of the problems, construct connections that are meaningful to them, and deepen their understanding.
In addition, allowing students to create their own strategies allows them the grace of trial-and-error. Recognizing mistakes and dead ends is an integral part of the learning process! Being able to recognize a personal mistake and adjust your process is much, much more impactful than simply being marked incorrect.
Have Children Reflect on What was Done and Why their Actions were Appropriate
A common strategy I often employed when studying at university was the “teaching” method. Once I felt I had mastered information, I would find a friend and attempt to “teach” them about the subject at hand. And, hey, there’s a reason this method works, and it’s beneficial to students of all ages!
After your children have invented a strategy and solved the problem in a way they feel is correct, have them put their process and solution into words. This reflection can be done through writing or talking. This reflection makes them organize and clarify their thinking so that they can communicate it clearly, which also deepens their understanding.
Provide Frequent Opportunities for Students to Share and Compare Solutions to Problems
After students have reflected on what they have done to solve the problem, they should share their process and answer with their peers. During this time, they will compare the strategies used and the resulting solutions. While both the process itself and, of course, finding the correct answer are important, that is not what this is about. Instead, it’s about helping students extend and expand what they already know so that they can learn more. Children will see there is more than one way to solve a problem, be validated, and build long-lasting confidence to smash those math anxieties. This also provides you with the opportunity to model any strategies you are familiar with.
While it can be difficult to relinquish instructional control or even to fit this in with the many, many other topics you need to cover, the impact and results will more than makeup for it. It’s not about the most efficient way to teach content, but the most effective way for children to learn it. This method of embedding problem-solving into our everyday curriculum beyond merely word problems is necessary for our children to learn the lasting skills they truly need to succeed.

Now, if you’re ready to test your newfound knowledge of problem-solving do’s and don’t’s, what better way than to toss some problems to your students? While you’re more than welcome to use the outline above in your next math lesson or elsewhere, another idea is to provide your children with STEM projects. STEM is engaging and covers a variety of subjects, but it can admittedly be intimidating, especially if you’re just starting out.
And, as always… Thanks for reading!