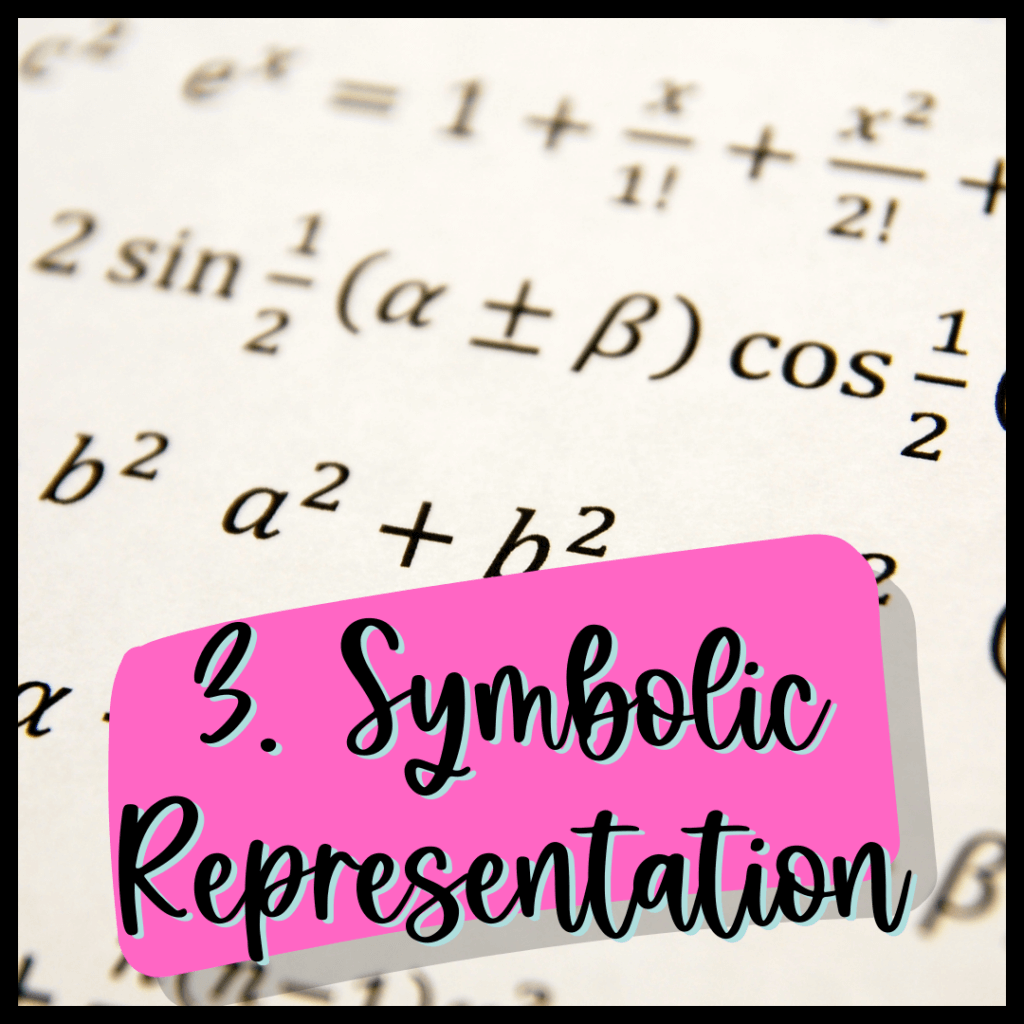Every day, you present new math ideas to students. From strategies to solutions to entirely new concepts, your kiddos can’t escape learning about math ideas on the daily… Not that they would want to, right? 😉
Joking aside, the truth of the matter is just that: some students would prefer to avoid learning new math ideas as much as possible. Math is quick to be branded in their little minds as “too hard” or “too confusing.” Even the most math-happy students can still experience burnout. Unfortunately, our modern-day classrooms don’t always allow much time to slow down, either–as educators, we’re pushed to get through the curriculum in a strict timetable with little opportunity to dwell on a topic if students are struggling.
In that case, it seems as though we’re better off if we preemptively head off problems before they begin. But how, exactly, can we do that?
Differentiation. You know, my all-time favorite word. I’ve only written, oh… Over a hundred blog posts about it!
Differentiation in math is a given, but how do we differentiate material when we first introduce a new math idea? After all, during the introduction phase, we don’t even know which students will struggle and how we can help quite yet. Well, as I mentioned earlier, we’re going to be preemptive about it! If we combine what we know about different learning strategies and the various ways to introduce new topics, then we can come up with several diverse methods to represent math ideas.
Let’s get started!
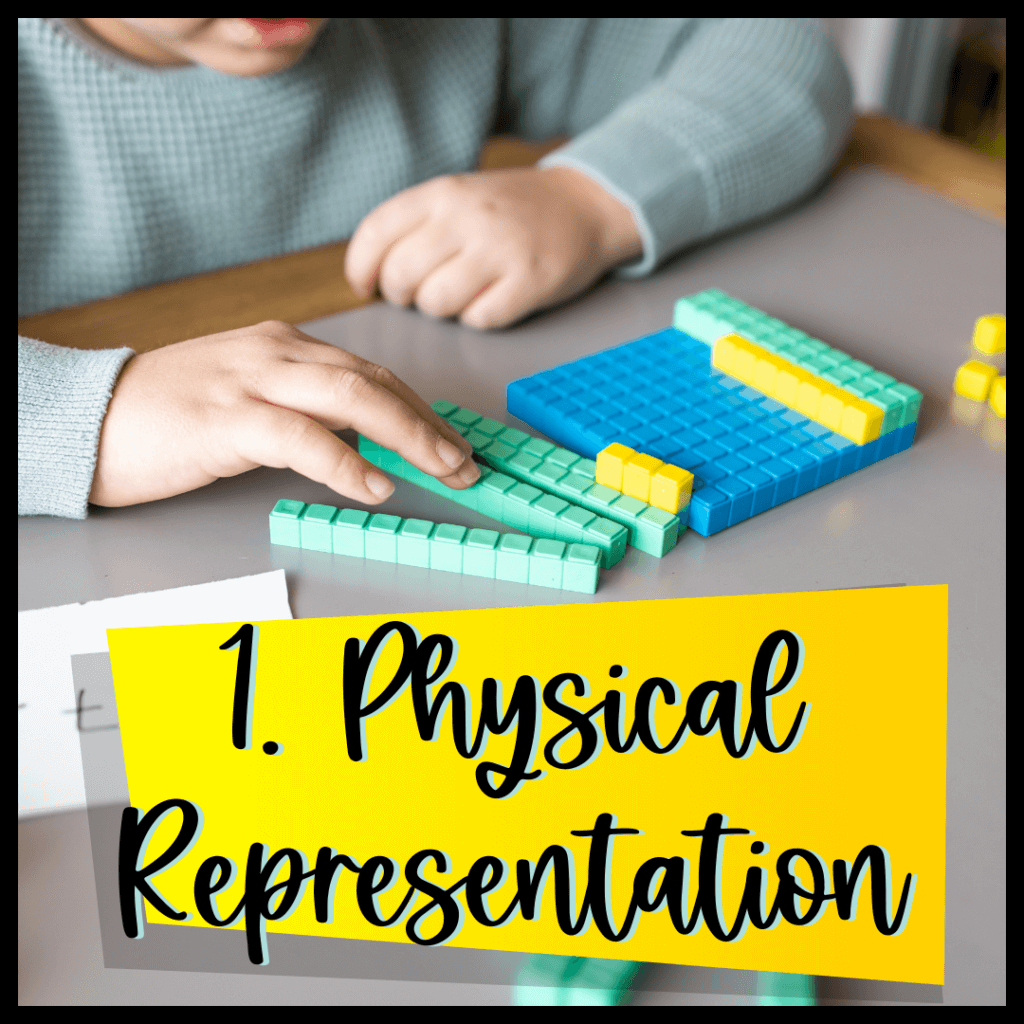
1. Physical Representation of Math Ideas
First and foremost, we’ll talk about my favorite method: physical representation of math ideas. Or, in other words, hands-on math! Anyone who knows me knows that I’m a huge advocate for hands-on learning, and for good reason–it works. It’s been shown time and time again that hands-on learning promotes a deeper understanding of math ideas by allowing students to actively engage with the concepts at hand, developing number sense and critical thinking skills.
At its core, physical representation in math refers to using tangible objects (manipulatives, base-ten blocks, etc.) to represent and explore concepts. When students use base-ten blocks to explore place value, or use fraction strips for fraction operations, the math ideas become “real” for them rather than an abstract notion that is difficult to comprehend.
In this way, physical interaction helps cement these concepts beyond rote memorization. We want students to truly understand math ideas–why strategies work and why they don’t, why a solution makes sense, and so on rather than simply memorizing numbers for the exam.
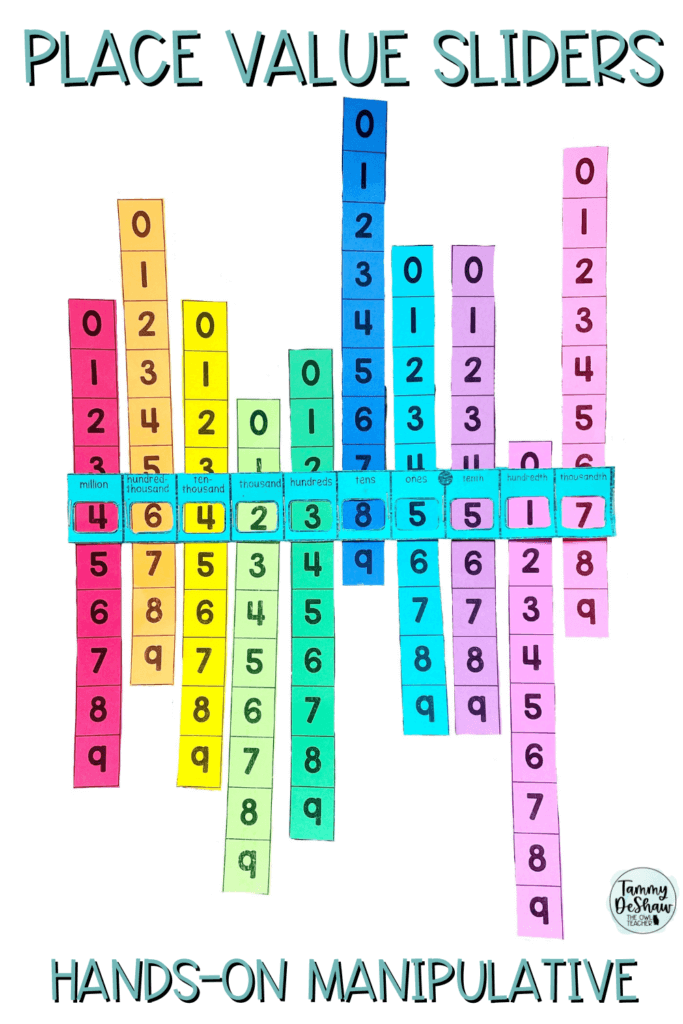
Most educators will have basic resources in their classrooms already for physical representation, such as base-ten blocks or pattern blocks. Others, such as fraction strips, can be created yourself with a little elbow grease. Even more resources are available online, such as my Place Value Sliders manipulative, and there are plenty more out there! You can find or create a physical representation for nearly all math ideas if creative enough.
2. Visual Representation of Math Ideas
While I adore physical representation in math, visual representation is certainly a worthwhile backup for those math ideas that are tricky to convey physically. Visual representation refers to the practice of using pictures, including graphs, charts, and diagrams, to represent a concept.
One of the most common examples of visual representation in the classroom is through number lines. For example, when teaching multiplication strategies, number lines are used as the foundation for skip counting. Another common example of visual representation is during your measurement unit–if your kiddos are anything like mine, they’re always engaged and captivated by all the colorful bar graphs!
As a baseline rule, when all else fails, I draw a picture, and I encourage my students to do the same. Providing students with something definite to picture when thinking over a math problem is invaluable. Similar to physical representations, visual representations of math ideas help make abstract concepts more concrete by providing students with a deeper understanding and, of course, developing number sense.
3. Symbolic Representation of Math Ideas
Symbolic representation is a natural part of the math-teaching process. Whenever you draw symbols on the whiteboard or include them on your worksheets, you’re using symbolic representation for math ideas. Symbolic representation is simply that–using symbols, such as numbers, plus signs, parentheses, and so on to represent an idea. When we write 2 + 2, our minds automatically translate the symbols to mean “two added to two.”
However, our students don’t have the decades of math knowledge like we do. When they first see these symbols, they look like a foreign language–because they are! Only through repeated experience can they learn this mathematical language of symbols.
Symbolic representation is vastly significant to laying a strong foundation for our elementary students. As they become fluent in mathematical symbols, students develop algebraic thinking–a much-needed tool for success in their later educational careers–and further their understanding of mathematical language as a whole. Due to its importance, it is essential to help young students understand symbols in math. If you’re interested in learning more, check out my blog post on the CRA (concrete, representational, and abstract) method.
A common strategy for teaching symbolic representation is to teach math ideas concretely at first, using visual and physical representations as mentioned previously. Once students have a sufficient understanding of the concept, then you may introduce symbols.
Keep in mind, however, that symbols are abstract. This means that students may struggle with comprehending them as well as they do concrete symbols. It helps to continue to remind students of concrete representations while moving through symbolic representation. Don’t be afraid to move back to physical and visual representations to clear up any confusion!
4. Linguistic Representation of Math Ideas
Similarly to symbolic representation, linguistic representation (sometimes referred to as “verbal representation”) is another facet of math ideas that we tend to implement naturally throughout our math lessons. Using phrases such as “greater than” to represent inequality and “sum” to represent the result of addition are all examples of linguistic representation of math ideas, and they go to show how common linguistic representation can be in our classrooms–certainly you’ve used these phrases at least once!
So how can we implement linguistic representation intentionally and in a manner that is most beneficial to our students? I think it bears repeating that, just like with the symbols of symbolic representation, the language of math is just that–an entirely new language to your students. Be conscious of the fact that your students are learning this new language!
In everything, repetition is key. While you shouldn’t necessarily start throwing huge words at your students, you also shouldn’t shy away from speaking this mathematical language altogether. At the risk of sounding like a broken record, you know your classroom best! Try to strike an even balance between challenging your students but not overwhelming them.
The significance of linguistic representation of math ideas stems from the fact that students should be able to verbally explain not only the math problem and solution, but also clearly communicate their thought process as they work through it. This is why I am a huge supporter of think-pair-share, especially in math! Just about every single math workshop unit of mine uses think-pair-share at some point, and for good reason. Verbally working through a problem with their peers helps to clarify students’ thinking processes and strategies. Regular use of think-pair-share is an excellent way to take advantage of linguistic representation.
Another easy way to implement linguistic representation in your classroom is to make it a habit to think out loud. When you are writing a problem on the whiteboard, drawing a picture, showing a strategy, or anything else, explain what you’re doing and why you know you’re doing it correctly. Alternatively, you can make mistakes on purpose, and ask students to share with a partner why they know you’re incorrect. Encourage students to use appropriate “math language!”
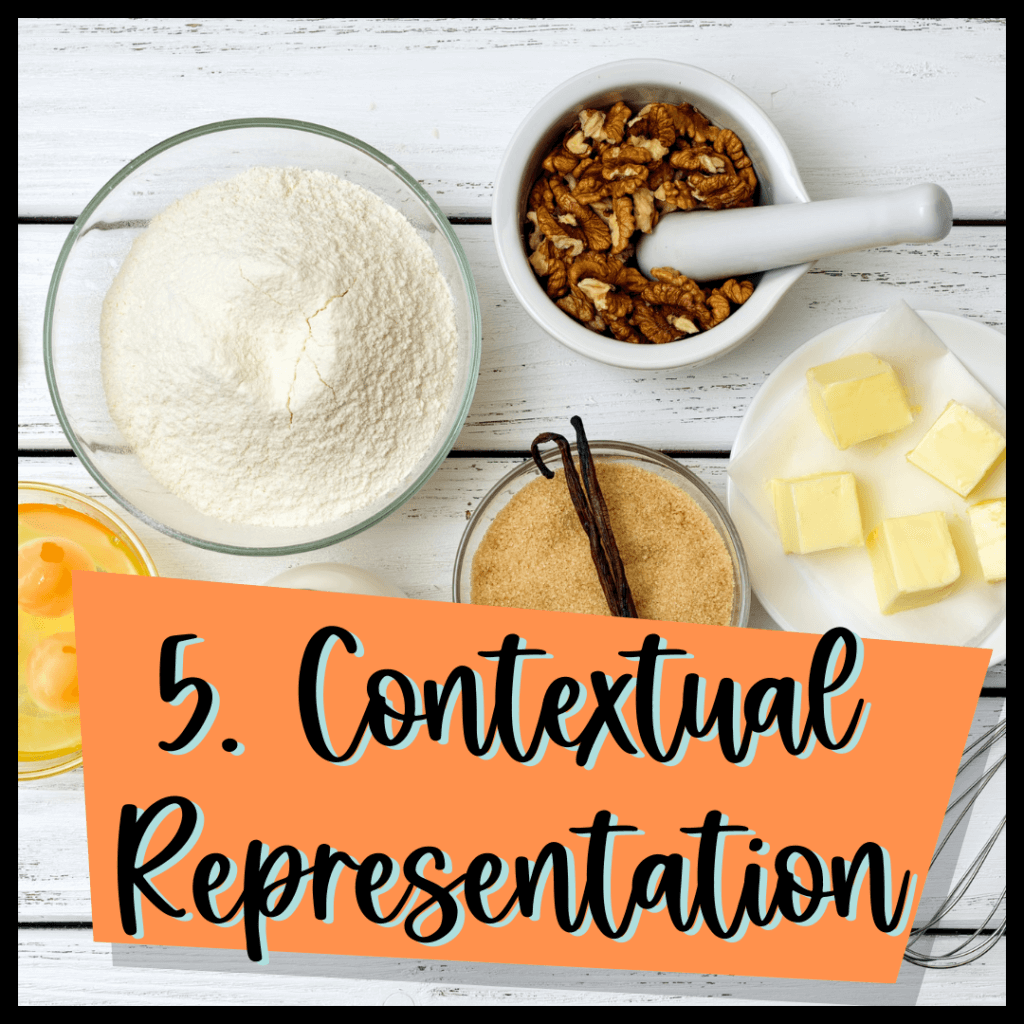
5. Contextual Representation of Math Ideas
Contextual representation of math ideas is the answer to the age-old question: “When are we ever going to use this in real life?” By providing real-world context to your math ideas, you can head off that question before it even pops up! In addition, including context helps students understand the importance of math ideas as well as making them more meaningful.
Some common examples of real-world context include connecting money to decimals, or connecting recipes to fractions. While there are plenty of examples of context you can provide for your students, it is also beneficial to have your classroom brainstorm their own examples. Encourage them to make their own contextual connections as a class–the more examples, the better, as students will be able to see how versatile and important math is in nearly every facet of life.
One of my favorite ways to provide contextual representation of math ideas is through project-based learning. From fraction feasts to building a town using area and perimeter, project-based learning provides real-world context to math ideas by showing students just how abundant math is! Like it or not, math is everywhere… So you may as well pay attention in class, right? 😉
By using a variety of these representations and forging connections between them, we as teachers can help students understand math ideas in multiple ways and support diverse learning styles. Differentiation is always the name of the game: students who struggle with one type of representation may be able to understand the concept more readily when presented with another type of representation.
Having a diverse learning environment is essential to enriching your students’ education and setting a strong foundation for their scholarly careers. Get students involved and engaged, and they’ll learn more than ever before.
That being said… Get out there and teach some math!