Geometry is a subject that can be fascinating for upper elementary students. But sometimes it can be challenging too. Some children can’t wrap their heads around the various polygons and their attributes. These polygon misconceptions can lead to frustration and confusion. This post is here to help you navigate the maze of polygonal misunderstandings and provide you with strategies to help your students build a strong foundation in geometry. Let’s get started on the ‘plane’ truth about polygon misconceptions and the strategies we can use to fix them.
The Polygon Misconceptions
2D shapes and polygons are the building blocks of geometry and can be downright dizzying trying to remember all the different vocabulary and attributes when you are young. It’s so easy for students to get tripped up on whether a shape is a rhombus or a kite or confusing congruence with similarity. While I’m sure I won’t cover all of the polygon misconceptions students have, I’m going to address the common ones I have seen (in no particular order) over my years of teaching.
1.) Is a rectangle also a square? Is a square also a rectangle? Argh!
If you have ever taught third-grade math, you know all about this one. Every year there are at least one (dozen) students who struggle with this. And every year that I taught this, I would be tempted to skip it because honestly, who cares! But I’m sure there’s a reason other than helping students learn how to categorize and classify based on the shapes’ attributes.
2.) Oh, that’s easy! That’s a rhombus!
Shapes, but most especially quadrilaterals can be identified wrong because of the way they are oriented on a page. Oftentimes, when students are given worksheets or shapes are drawn on anchor charts, they are drawn in the same way that we are most familiar with. Therefore, when a square (for example) is shown tipped on a corner, students automatically assume it must be a rhombus rather than looking at its sides and the angles to determine which shape it really is.
3.) It has the same number of sides so they are definitely congruent!
Children struggle with the difference between similar shapes and congruent shapes A LOT. Sometimes it’s the idea that if the two shapes have the same number of sides, then they must be congruent. Another issue is that sometimes students look at shapes that look alike but are different sizes and assume they are congruent because it’s the “same shape.” Lastly, if the orientation of one of the shapes is different from the other, but exactly the same size and shape students may believe that one is a completely different shape.
4.) Wait, this 3D shape is the same shape as this 2D shape? You’re kidding!
This was a struggle I had when I was younger myself. How could a cube possibly be a square? I mean, they look completely different. Then it was okay, okay. I see several squares put together to make a cube, but that doesn’t mean it’s a square. It really took me a while to grasp that it was the same shape but with an extra dimension. So, keep in mind you may have a few little ones in your class thinking you’re nuts when you say it’s the same shape.
Suggestions to Fix the Polygon Misconceptions
These ideas below apply to virtually all the polygon misconceptions above, but some may be more specific. I didn’t include the more obvious ones such as determining the background knowledge of the student, the need to scaffold, the need to regularly re-evaluate what the student understands. Instead, I’m suggesting some intervention ideas to help them break through misconceptions. Ultimately, providing ample opportunities to practice identifying the different polygons and their attributes will help solidify their understanding and correct their misunderstandings.
1.) Yep, you knew it. Let’s make it hands-on.
Of course, I was going to say making it hands-on. That’s like my motto, right? Giving students as many opportunities as you can explore the attributes of shapes in a tactile way will help with polygon misconceptions way more than you think. Instead of always using the same manipulatives or tools, consider mixing things up a bit. Provide multiple opportunities but with a different tool or manipulative each time. For instance, students can use geoboards, pattern blocks, or tangrams. If you have straws and twisty ties lying around, you could have students create polygons. To do this, just have students insert one end of a twisty tie into the end of a straw and the other end of the twisty tie into another straw so that you have a “flexible” angle. Then do this again with the other end of the straw connecting all the twisty ties and straws. The twisty ties form the angles and the straws are the sides. This makes it easy for students to move the sides around to form other polygons and to see the relationships.
2. Since we are discussing hands-on manipulatives, let’s throw visual aids in the mix.
Using the straws and twisty ties above to create hands-on polygons that are easy for students to manipulate is a great tool, but sometimes you can’t always bring out manipulatives. When this is the case, reach for the visual aids. They are the next best thing!
Creating pictures, using diagrams, using graphic organizers, watching videos, and other real-life examples are helpful for all students, not just visual learners. In fact, Mash Up Math creates a diagram for students illustrating the relationship between squares and rectangles using a cookie analogy. This is definitely a great way to help students understand how a square is also a rectangle but a rectangle is not a square. In this image that I “borrowed” from that website (click here to visit that blog post), you’ll see how it helps students understand that all squares are rectangles, but not all rectangles are squares just like all Oreos are cookies, but not all cookies are Oreos. Visual aids can be powerful.
3.) Let’s face it, geometry can be vocabulary heavy!
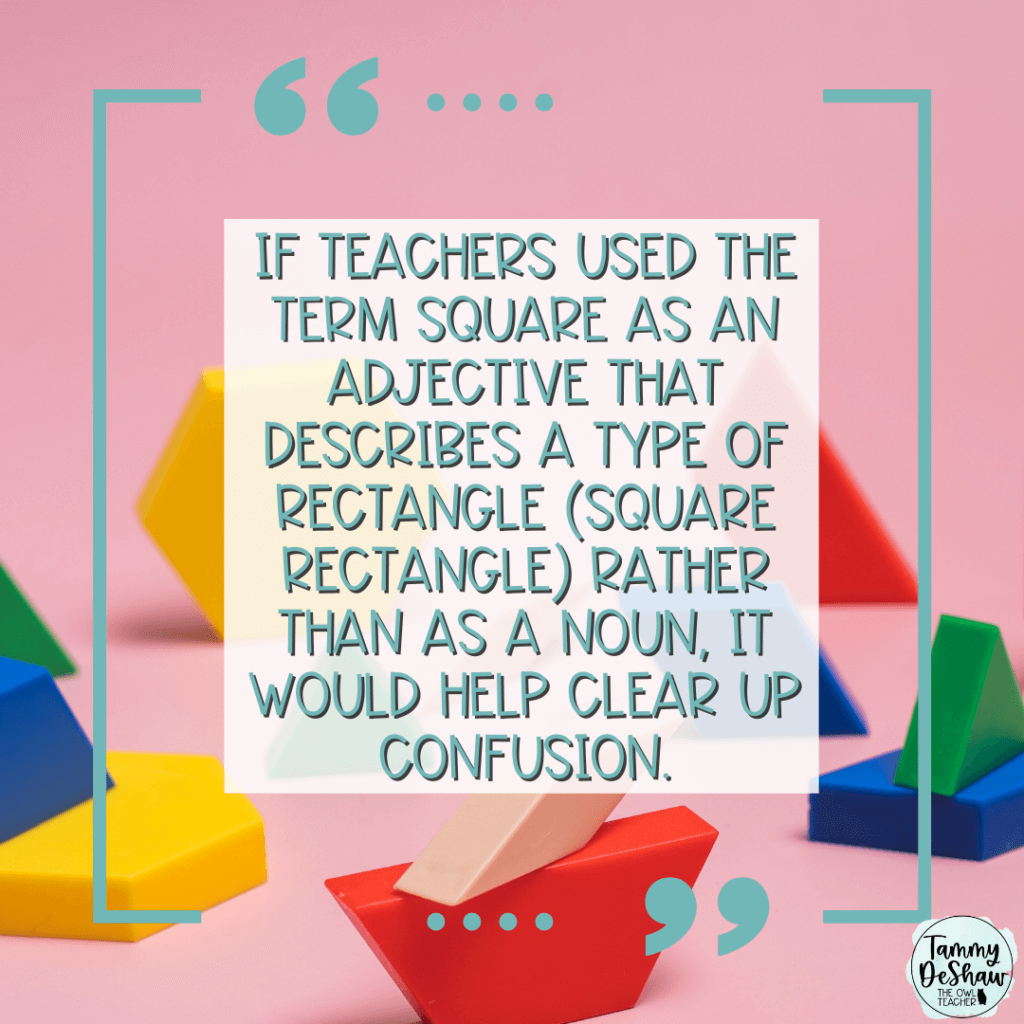
It’s easy to breeze through geometry using all the different terms without even thinking about it. However, to most children in elementary school all the vocabulary terms can be very overwhelming. It’s almost a foreign language to them. With that said, we need to teach the vocabulary terms during our polygon lessons (or any geometry lesson) explicitly – as if all our students are English Language Learners. Think about the language you are using. For instance, did you know that we should be using the term square as an adjective rather than a noun? A square is a word that describes a type of rectangle, isn’t it? That would make it an adjective AND couldn’t we say this is a square rectangle when we are discussing the well-known polygon (described as a noun) square? If teachers regularly used the term square as an adjective that describes a type of rectangle (square rectangle) rather than a noun, it would definitely clear up some polygon misconceptions!
4.) Hold on! Think about your thinking when you think!
I love saying that. It’s a great way to encourage metacognition in students. If we can get students to slow down and really reflect on what is happening, what they are thinking, what they have learned, and what they still need to learn, this can help them improve their understanding. Often times what students are thinking or saying doesn’t even make sense and once we force them to slow down and think about what they are saying (or thinking) they notice it. Other times there are students who just want you to do the work for them. We need to teach them to be problem solvers and really think about it.
5.) Bring on the tech.
There are lots of technology tools such as interactive whiteboards, tablets, online math games, and platforms such as IXL that are engaging and can help students visualize math concepts. Some even differentiate content for students so that they can have a more personalized experience that can address their individual misconception. These platforms (I’m not an affiliate) can also be helpful for students who need extention activities to challenge them.
There are always going to be students who misunderstand math concepts and there very well could be some that aren’t listed here. These listed are the common ones I have seen and are to help you know what to watch for. While I’m sure there are plenty of other strategies and intervention ideas to help students that have polygon misconceptions, these should help get you started to address them.
Good luck getting these misconceptions untangled!